In any endeavor it’s important to know why you’re doing what you're doing. This is no different. To the unpracticed eye, addition and subtraction problems are pretty much the same, although they may be phrased slightly differently from time to time. However, I’ve come to learn that these differences are really huge in the minds of my young math students and absolutely crucial to for me to understand in order to perceive of what my students are thinking!
more from Sue
|
The essential elements of addition and subtraction:
|
|
| Addition/Subtraction Concept |
Example of Math Problems Related to Concept |
Also consider... |

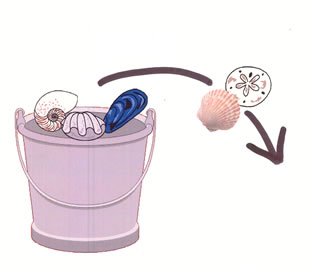
increasing
|
Basic: I had three shells in my bucket. I put two more shells in my bucket. How many shells are in my bucket now?
number model: 3 + 2 = ___
Intermediate: Twenty-five children are riding on the bus. At the next stop, 5 more children get on. How many children are on the bus now? |
This concept can also be framed as "change to more." The Univ. of Chicago Math Project diagrams the concept this way:
important variation... |
decreasing
|
Basic: I had five shells in my bucket. I threw two shells back into the bay. How many shells are in my bucket now?
number model: 5 – 2 = ___
Intermediate: A bus leaves school with 35 children. At the first stop, 6 children get off. How many children are left on the bus?
number model: 35 – 6 = ___ |
The situations to the left can also be considered "change to less" problems and diagrammed as:
important variation... |
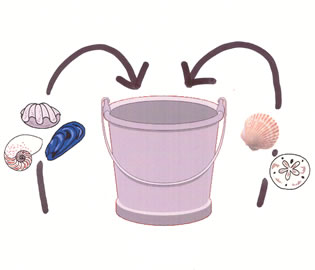 combining combining
|
Basic: I put three shells in my bucket and my brother put two shells in my bucket. How many shells are in my bucket now?
number model: 3 + 2 = ___
Intermediate: Twelve fourth graders and 15 first graders are on the bus. How many children are on the bus?
number model: 12 + 15 = ___
|
This raises the idea of total. We can frame this concept by calling it "parts and total" as diagrammed here with the bus problem's data:
|
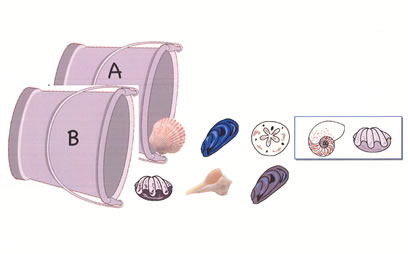
 separating separating
|
Basic: I have five shells in my bucket. Three of them are mine, and the rest of them are Bill's. How many of the shells belong to Bill?
number models:
5 – 3 = ___
3 + ___ = 5
Intermediate: Thirty-five children are riding on the bus. Twenty of them are boys. How many girls are riding on the bus?
number models:
35 – 20 = ___
20 + ___ = 35 |
Here is a similar problem, but the total is known and one of the parts is unknown.
important variation... |
 comparing comparing
|
Basic: Bill has three shells in his bucket. I have two more shells than Bill. How many shells do I have in my bucket?
number model: 3 + 2 = ___
Intermediate: I know that I am four inches taller than my younger brother. If he is 41 inches tall, how tall am I?
number model: 41 + 4 = ___ |
What is so different here is that we are now working with two quantities! In this particular comparison situation, the smaller quantity and the difference are known:
important variation... |
 finding the difference finding the difference |
Basic: I have five shells and Bill has three. How many more shells do I have than Bill?
number models:
5 – 3 = ___
3 + ___ = 5
Intermediate: There are 12 fourth graders and 8 third graders. How many more fourth graders are there than third graders?
number models:
12 – 8 = ___
8 + ___ = 12 |
Most comparison situations are not like the one shown above. Instead, we are more often determining the difference between two quantities:
|
Liping Ma organizes problem types in such a way that addition serves as a bridge to subtraction.
|


 combining
combining separating
separating
